Safety Evaluation of Li-ion Batteries

Research

Energy storage systems (ESS) such as Li-ion Batteries (LIBs) are the solution for many applications including cellphones and electric vehicles. However, they can pose serious hazards if their safety is compromised such as after sustaining mechanical damage. Lithium-ion batteries have several internal processes that contribute to their response to current excitation. There is a frequency range associated with each of these internal processes in which they are most active. Therefore, conducting EIS (Electrochemical Impedance Spectroscopy) experiments can be used to investigate the effect of different excitation/environmental conditions on these time constants. EIS is an important tool in analyzing and modeling ESS which is based on applying sinusoidal inputs in the form of voltage or current to the cell and measuring the output current or voltage. EIS plots provide the response of the system to a wide range of frequencies. However, due to the presence of several processes inside ESS, the interpretation of EIS data is a challenging task. Therefore, supplementary tools are needed to extract the required information from EIS data. Distributed Equivalent Circuit Model (DECM) and Distribution of Relaxation Time (DRT) are two main tools for analyzing EIS data. However, in the DECM approach, the number and type of circuit’s elements and their connection to the cell’s physics is still an open question. DRT approach can decouple the processes by identifying the time constants of the EIS data. However, the DRT method and its variations do not work on ESS due to their complex impedance spectra, which violates the underlying assumptions commonly used in DRT.

 

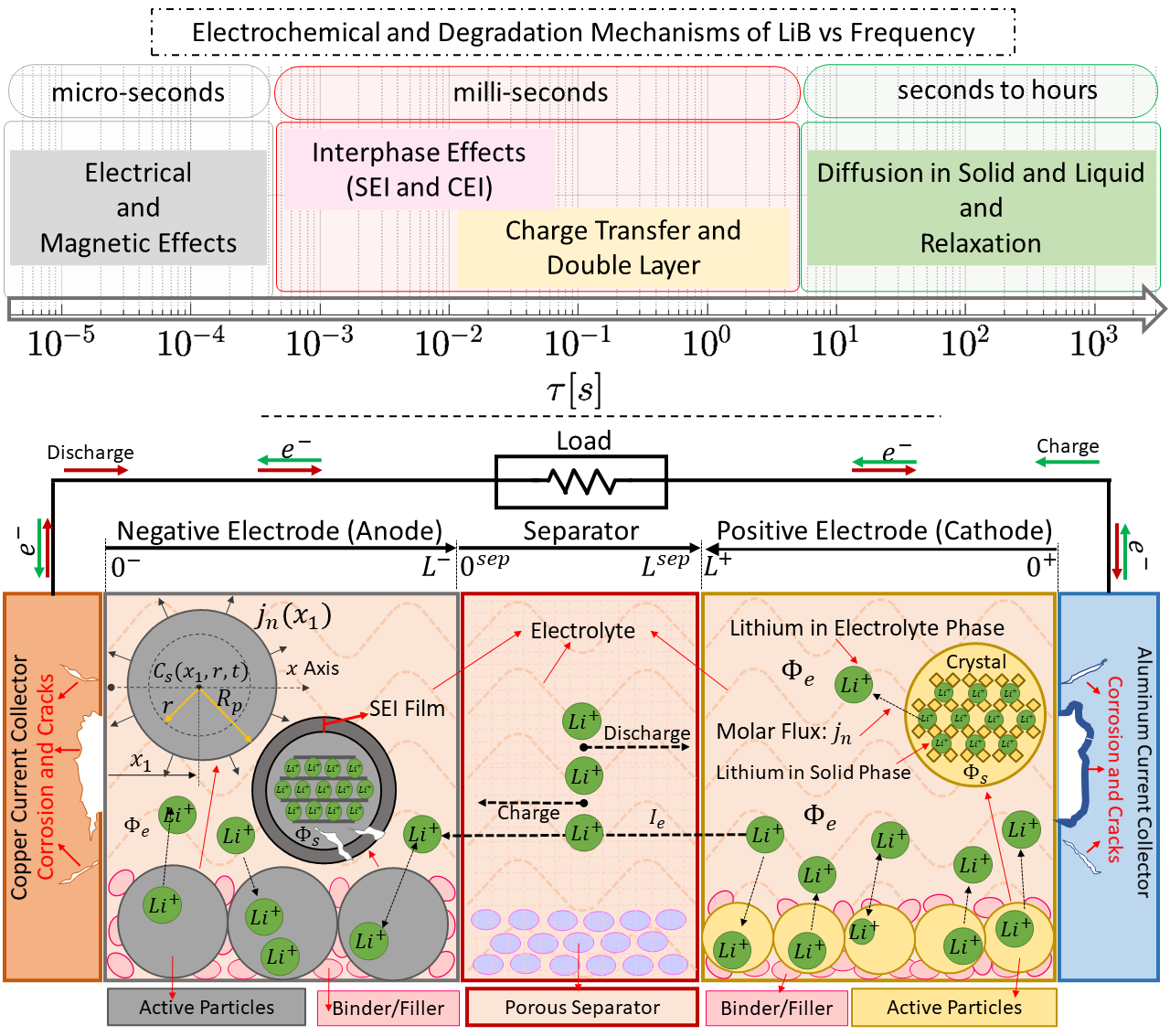
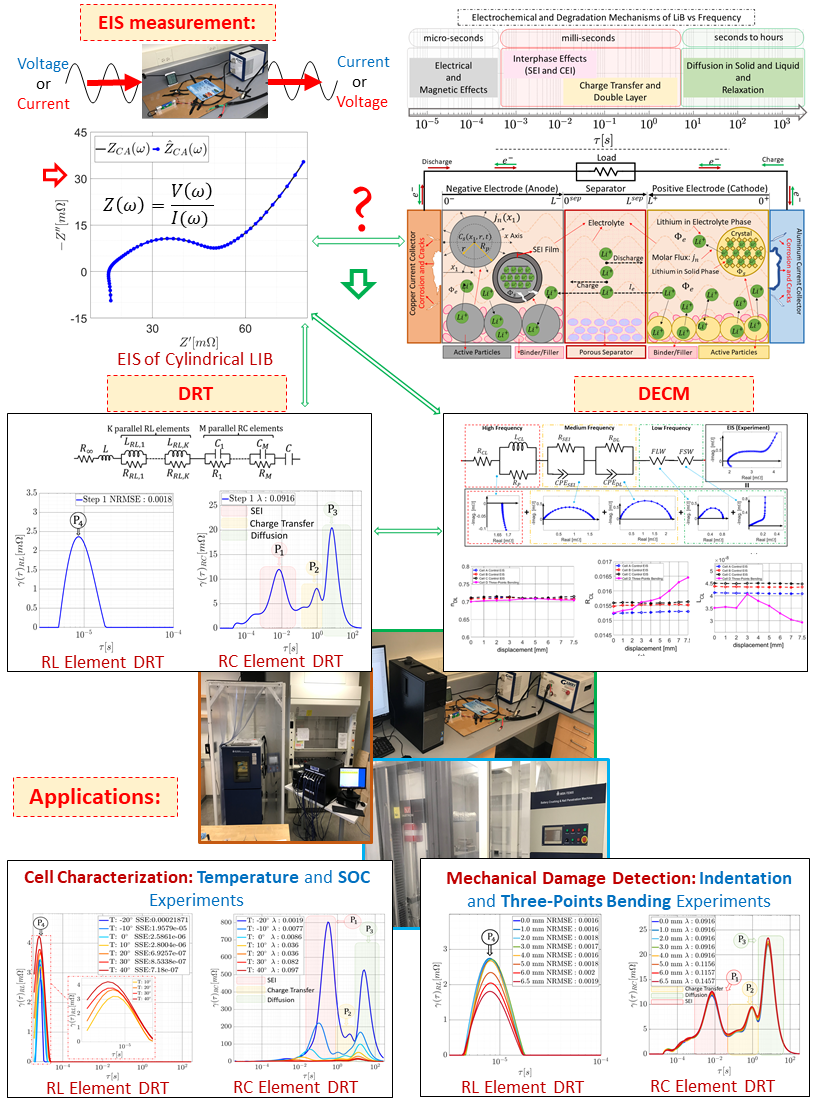
Schematic of Li-ion Battery components with different Electrochemical and degradation mechanisms vs Frequency.

In this research, we address characterizing the safety status of Li-ion batteries based on their time constants. We introduce a method to determine time constants of energy storage systems (ESS) using their impedance spectra. The Distribution of Relaxation Times (DRT) function has been suggested to determine such time constants. We formulated DRT as a ridge regression optimization. We introduced criteria (discrepancy, cross-discrepancy, and the normalized root mean squared errors) to determine the time constants as the peaks of the resulting distributed function. The proposed approach was validated using experiments on Li-ion batteries (LIBs). We measured the impedance spectra of LIBs using an EIS instrument at different cell temperatures ( to and State-of-charge ( and SOC). We determined the time constants and used the SOC and temperature data to assign the peaks to appropriate electrochemical processes and verified them using the experimentally calculated time constants reported in the literature. We hypothesize that the time constants of ESS can be used for their fault detection and health monitoring, such as after sustaining mechanical load/impact. To validate this hypothesis, we measured impedance spectra and determined time constants of intact and mechanically damaged 18650 cylindrical cells. The DRT peaks showed the main differences between these groups and suggested criteria to determine the extent of mechanical damage from the impedance spectra. The method has application in the fields beyond ESS, where the frequency response can be measured. The time constants determined using our proposed method can guide the control-oriented data-driven models as well as the equivalent circuit models of ESS. For example, the number of time constants can determine the minimum number of elements to model ESS as well as the range of frequency at which these elements are excited. Furthermore, the time constants (and the basis function) can be used for distributed modeling of ESS with many elements and used for their fault detection and health monitoring.

Publications:

  • Derakhshan, M., Sahraei, E., and Soudbakhsh, D. “Detecting Mechanical Indentation From the Time Constants of Li-ion Batteries, ” in Cell Reports Physical Science, 2022, doi: 10.1016/j.xcrp.2022.101102.
  • Derakhshan, M., and Soudbakhsh, D. “Temperature-Dependent Time Constants of Li-ion Batteries,” in IEEE Control Systems Letters, vol. 6, pp. 2012-2017, 2022, doi: 10.1109/LCSYS.2021.3138036.
  • Keshavarzi, M., Derakhshan, D., Gilaki, M., L’Eplattenier, P., Caldichoury, I., Soudbakhsh, D., and Sahraei, E., “Coupled Electrochemical-Mechanical Modeling of Lithium-Ion Batteries Using Distributed Randle Circuit Model,” 2021 International Conference on Electrical, Computer and Energy Technologies (ICECET), 2021, pp. 1-6, doi: 10.1109/ICECET52533.2021.9698796.
  • Derakhshan, M., Gilaki, M., Stacy, A., Sahraei, E., and Soudbakhsh, D. (January 22, 2021). “Bending Detection of Li-Ion Pouch Cells Using Impedance Spectra.” ASME. Letters Dyn. Sys. Control. July 2021; 1(3): 031005. doi: 10.1115/1.4049527.
  • Soudbakhsh, D., Gilaki, M., Lynch, W., Zhang, P., Choi, T., Sahraei, E. Electrical Response of Mechanically Damaged Lithium-Ion Batteries. Energies 2020, 13, 4284. doi: 10.3390/en13174284
  • Stacy, A., Gilaki, M., Sahraei, E., and Soudbakhsh, D. ”Investigating the Effects of Mechanical Damage on Electrical Response of Li-Ion Pouch Cells,” 2020 American Control Conference (ACC), 2020, pp. 242-247, doi: 10.23919/ACC45564.2020.9147883.
  • Sahraei, E., Gilaki, M., Lynch, W., Kirtley, J., and Soudbakhsh, D. “Cycling Results of Mechanically Damaged Li-Ion Batteries,” 2019 IEEE Electric Ship Technologies Symposium (ESTS), 2019, pp. 226-230, doi: 10.1109/ESTS.2019.8847923.

Leave a Reply