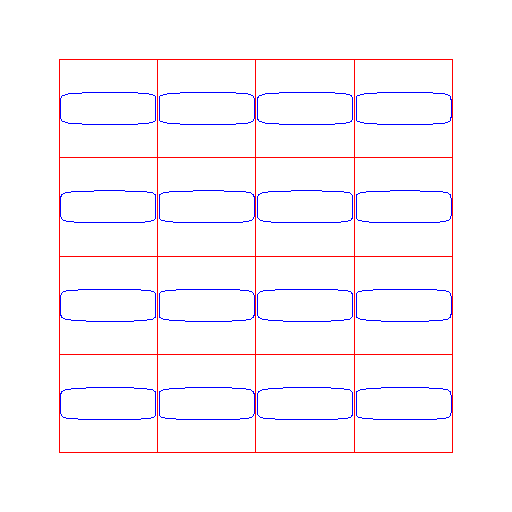
The Vigdergauz shape is a two-parameter shape of an inclusion in a periodic composite that has many optimal properties. It minimizes the stored elastic energy, maximizes stiffness, minimizes stress concentrations. The Vigdergauz shape has an explicit parametrization in terms of elliptic integrals. This allows one to plot it exactly, as one would plot a circle.
Here the Vigdergauz microstructure with volume fraction 0.3 adjusts itself to the changing average strain.

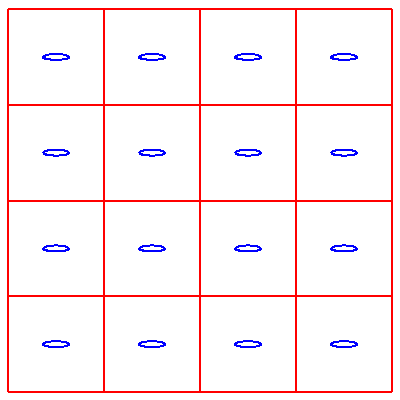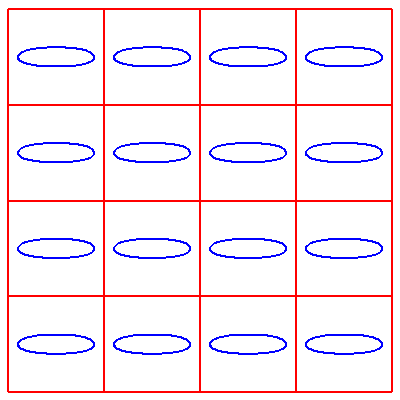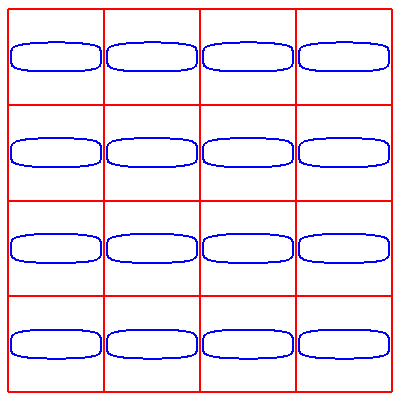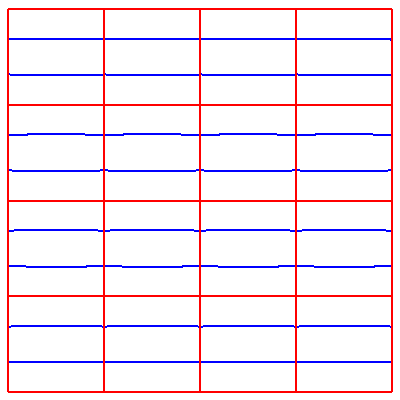
Here we have the growth of the the Vigdergauz inclusion from nucleation as an Eshelby ellipse to the layered microstructure under the constant average strain over the period cell.
When the average strain reaches a critical value the Vigdergauz microstructure degenerates into layers.